
Mathematics
Education
EMAT 6680,
Professor Wilson
Final Assignment by Ursula Kirk
A.
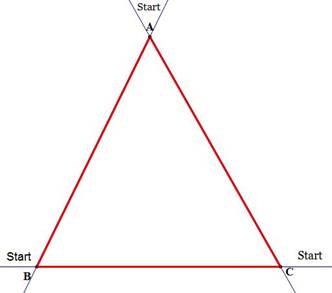
Bouncy Barney
Barney is in the triangular room shown here. He
walks from a point on BC parallel to AC. When he reaches AB, he turns and walks
parallel to BC. When he reaches AC, he turns and walks parallel to AB. Prove that Barney will eventually return to his starting
point.
How
many times will Barney reach a wall before returning to his starting point?
Explore
and discuss for various starting points on line BC, including points exterior to
segment BC.
Discuss and prove any
mathematical conjectures you find in the situation.
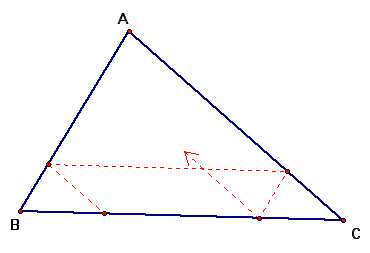
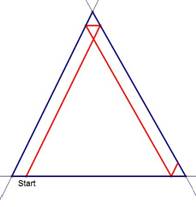
Barney’s Path
Here is the path
that Barney takes when he starts from the STAR point on the picture. He comes
back to his starting point after 6 steps.
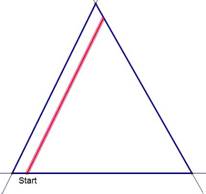
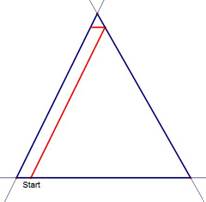
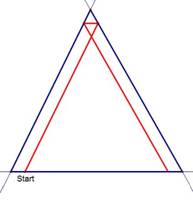
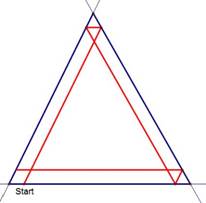
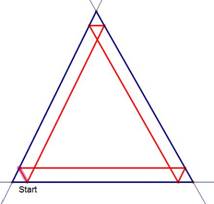
There are three points from where Barney
will be able to complete his route in only 3 steps. If Barney starts from any
of the middle points of the sides of the triangle, he will be done in 3 steps.
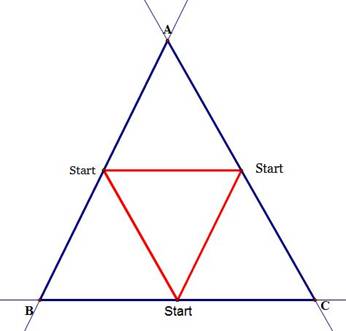
Barney will be done in 3 steps also, if he starts from any of the
vertices of the triangle.
If he starts
from a point outside the triangle, he will be done in 6 steps. From V, W, X, Y,
Z or U, Barney will be back to the starting point in 6 steps.
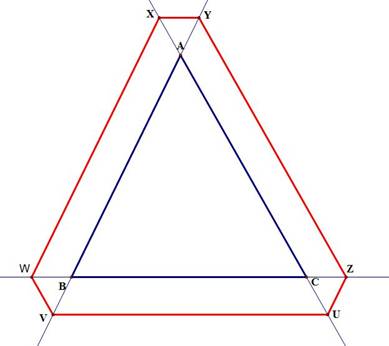
Therefore, my conjecture is that Barney will always complete his route
in 6 steps with the following exceptions:
·
Barney
starts from any of the vertices of the triangle; in this case Barney will
complete the route in only 3 steps.
·
Barney
starts from the middle point of any of the sides of the triangle; in this case
he will be done in 3 steps.
B.
Complete a Write-up on your Web Page for
one additional investigation, chosen from Exploration 0 through Exploration 12.
·
I have chosen to
complete my write up from exploration 0, investigation 7.
Graph the equation:
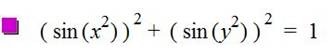
Where n=1
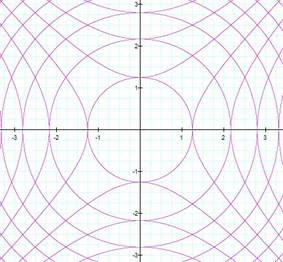
Our graph at n=1 has
circles with centers at (0,0). The circles get closer together as we approach infinity.
Also, we have vertical and horizontal hyperbolas symmetric about the x-axis and
the y-axis. Like the circles, the hyperbolas are getting closer as they move
away from the center.
As we change the value
on n, our graph also changes. As n approaches 0, we start getting bigger gaps
in between the graphs. The circles and the hyperbolas are gone. Still our graph
is center at (0,0) and it is symmetric about the x-axis and the y-axis.
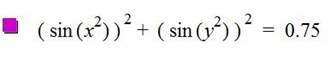
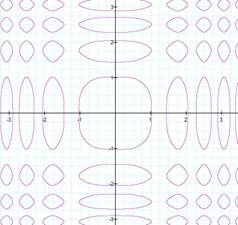
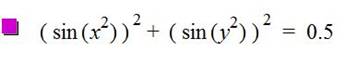
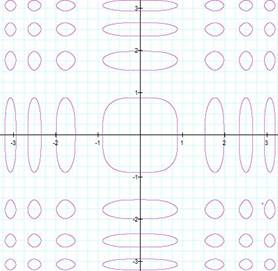
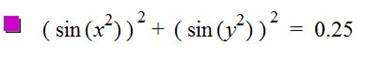
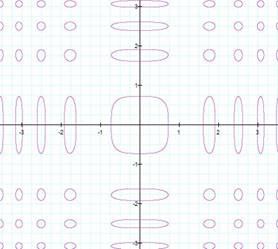
Finally when n=0, the
graph is totally gone.
If we start increasing
from 1 to 2 the gaps start getting also smaller and at n=2 the graph is totally
gone.
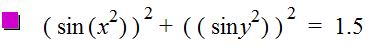

Here, we do not have a
center piece any more. The shapes have change, but still the graph is symmetric
over the x-axis and the y-axis.

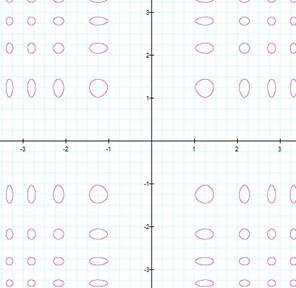
As we get closer to 2,
the gaps are getting bigger and the graph is disappearing.
Here is a movie for the
equation when n varies from 0 to 2
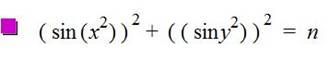
MOVIE